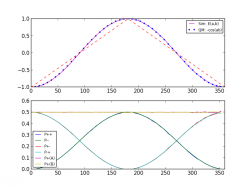
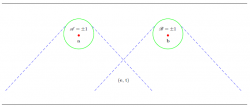
James.
"I don't say prior anything exists priorly as if the future is active."
What does that mean?
"I say the means for the end exists before the end occurs. Use the word probability or whatever, but it has no mystical connotations to it."
No? Then all you're saying is that the likelihood of anything happening at any moment that it happens is ordained by the initial cosmological condition. I agree. Now explain it without invoking a mystical cause. Contrary to your opinion, we do get cause "for free." Life is free.
"Your repeated efforts to define your beliefs as resulting from rational thinking is betrayed as false by your adoption of theory."
Not my adoption. It's what "rational" means.
"What I mean by empirical is empirical evidence. What I mean by rational is to avoid empirically unwarranted inventions and learn that which it is that empirical evidence is communicating to us."
And that requires a theory. Otherwise, your belief is a "just so" narrative, like any religious narrative. There's nothing wrong with that -- you just can't call it science.
"Tom: "No such correspondence exists."
Me: The correspondence that I use exists."
Yes, but it's a correspondence of belief to evidence; in other words, if one believes that witches float, every prosecution of an accused witch in medieval times was just.
In the same vein, if one accepts probabilism as fundamental, every experimental violation of Bell's theorem confirms it.
"Skip Your continuation ...'The probability is a myth, ... you do not differ.'
Me: "You can't see yet where I differ. You don't yet understand what I describe to you. Theory isn't the tool you need."
Theory is the only *objective* tool you have.
Tim: 'True determinism is as you claim -- based in the outcome of physical events, empirical. If those events are probabilistic, however, what you claim actually opposes what you believe.'
"Me: That statement demonstrates that we are still not communicating. You obviously mean something different with 'probabilistic'."
How many meanings do you think that term can have? It's quite unambiguous.
"I presume that you are thinking in terms of some probability theory. Theory isn't the tool you need. Theory is the practice of inventing substitutes to serve in place of the unknown in physics equations. You won't need those invented properties if you learn from empirical evidence. The first corrective step you need to take is to recognize that mass should not have been declared a fundamental indefinable property.
Tom: "Will you be a believer, or a scientist?"
Me: This statement is similar to many self promoting statements that you make. For you theory rules. You believe it and more problematic is that you preach it."
I sure do. Not for my sake -- for the sake of rational thought. Too many witches have been thrown in the river by believers.
Best,
Tom