Classical Spheres, Division Algebras, and the Illusion of Quantum Non-locality:

Akinbo,

You are misunderstanding. Joy's work supports EPR and opposes the idea that quantum mechanics is a complete theory. In fact, it is quite easy to show that QM is incomplete. The real foundational question is whether reality is inherently probabilistic ("God plays dice") or determined by hidden variables imposed by nature independent of the observer.

So far as the correspondence of points and lines, these are basic theorems in geometry. There are as many points in this line: ___, as there are in the entire universe.

Best,

Tom

Tom,

As I posted I am in support if 'Joy's work supports EPR and opposes the idea that quantum mechanics is a complete theory'. The support is however qualified so that we don't substitute one incomplete work with another. For example, I have suggestions on what can be HIDDEN, LOCAL, VARIABLE and REAL that Joy can investigate. That way, a local hidden variable theory can explain the incompleteness of QM. Now we may not be able to identify this agency if as you say, we rely on the premise that "There are as many points in this line: ___, as there are in the entire universe".

How many of these your lines ___ ___ ___ ___ ___ are in this message box and how many of this message boxes are in your line?

If your line and the message box contain the same number of points, then you should be able to fit the message box into your line topologically, talk less of letting your line contain the universe.

Regards,

Akinbo

Akinbo,

"The support is however qualified so that we don't substitute one incomplete work with another."

Rest easy, then, because relativity -- the special and general theory -- is mathematically complete.

I am not going to discuss the point-line thing in this forum. It's well understood geometry.

Best,

Tom

Rather than being quite so dismissive..

I might recommend a look at what Atiyah and Sutcliffe have to say about Point Particles, in the attached paper. Besides; the point to consider here is the one at infinity that, as you love to remind us Tom, is a defining element of projective spaces. The magical thing about parallelized S3 and S7 is that they connect proximal and distal space seamlessly. So the difference between collinear and non-collinear points is an essential and sometimes subtle distinction - that is certainly germane to this discussion. Does topological space have thickness? Approaches to quantum gravity with this feature have been suggested (e.g. - Arkani-Hamed, Dvali, Dimopolous).

But as for the question of infinite divisibility, people tend to believe Dedekind without fully understanding what his method was about, or trying to understand, and just consider the matter settled. Certainly though; the experts have been around the block with this issue, and the consensus is that ANY finite span on the number line DOES contain an infinite range - in that it is endlessly sub-dividable. The real question (pun intended) is whether nature offers us an unbroken span. I think Joy's topological approach may absolve us of the need to quantize space, in order to reconcile things, so that is the question here.

Regards,

JonathanAttachment #1: 0105179.pdf

Thanks Jonathan for the journal reference, although my mathematical credentials are not excellent.

You say, "I think Joy's topological approach may absolve us of the need to quantize space, in order to reconcile things,...".

I beg to disagree, while a topological approach may help, Joy's approach MUST NOT shy away from its clear responsibility having started his work with a statement like... "The Point Bell Missed" and "Although lines and planes contain the same number of points...".

Firstly, this statement admits that lines and planes consist of points.

Secondly, by saying the points are numbered is to say they are discrete. Only discrete things can be numbered.

Thirdly, a line is claimed to be 1-dimensional and a plane 2-dimensional. How is a 0-dimensional object contained in a 1-dimensional one? Are the points in a line and those in a plane of same dimension?

Fourthly, the claim that lines and planes contain the SAME number of points needs to be clarified. Is it supportive of what Tom said that "...There are as many points in this line: ___, as there are in the entire universe" and "I am not going to discuss the point-line thing in this forum. It's well understood geometry". What Tom is saying is that number of points on the line is infinite and the number in the universe is infinite, so both the line and the universe contain the SAME number.

The question I would have asked Tom, but can't since he says for him the case is closed is, whether his line, ___ and a segment of it also contain the SAME number of points. If so, whether points can then be said to be capable of being counted as to make assertions like two geometrical objects having the same number as Joy started with.

Jonathan, henceforth we must demand strict definition of what anyone, particularly the more mathematically inclined are asserting. It is from not being as demanding that mathematicians were allowed to introduce 'a line having length but with a breadth of absolute zero' and a 'surface of absolute zero thickness' into our physics.

Akinbo

Jonathan,

I always appreciate your ability to get to the nub of the issue. "I think Joy's topological approach may absolve us of the need to quantize space, in order to reconcile things, so that is the question here."

Indeed, I think so as well. I was disheartened that Vesselin Petkov's entry a couple of essay contests ago ("Can spacetime be quantized?"), didn't get near the attention it deserved.

At the end of the day -- at a foundational level -- we can discard the idea of particles, though we cannot discard the continuum.

The Dedekind cut principle is one my favorite mathematics results, and I agree with you that it is ill understood, even often misinterpreted. Dedekind says that, e.g., there exist two numbers whose product is sqrt2, even though one cannot provide a procedure for multiplying the two. By existence, however, because sqrt2 is an algebraic number, we are assured the computability of corresponding points by which 2(sqrt2) sets an upper bound of correlation . The current proliferation of computer simulations of Joy's framework is rich confirmation. That's only the beginning -- aside from mere computability, Joy's research promises to tell us what "quantum" really means.

All best,

Tom

I was not surprised to see Matt Leifer's entry win first place in this year's FQXi essay contest. Yes, it's a good essay, well argued and worthy of a contest prize.

More important to me personally, however, is that it represents what I observe is a longtime Perimeter Institute and FQXi bias toward probability models based on Bayesian philosophy. For those not familiar with the difference between what are called Bayesian and Frequentist models -- a Bayesian assumes a definite probability on the interval [0,1], requiring a measure of personal belief in the correct probability outcome for a given problem. A frequentist model is objective, based on the average of a sufficient number of independent Bernoulli trials (throws of the dice) such that one's confidence in the correct probability for a given problem increases with the number of trials.

My reaction to Matt's conclusion that "My main argument is that, on the subjective Bayesian interpretation of probability, 'it from bit' requires a generalized probability theory" alternates between "of course," and "so what?"

The fact is, that a generalized probability theory based on Bayesian principles is oxymoronic. One gets from it what one assumes in the first place, and cannot get otherwise. So to conclude, "A subjective Bayesian analysis of noncontextuality indicates that it can only be derived within a realist approach to physics" is simply saying that realism is in the mind of the observer and nowhere else. In no philosophy except standard quantum theory is "realism" defined this way.

"At present, this type of derivation has only been carried out in the many-worlds interpretation," says Matt. Either he misunderstands what Everett's interpretation is actually saying, or he is trying to co-opt many-worlds to coat his theory in a pseudo-objective patina. The fact is, there is no collapse of the wave function in Everett's interpretation; therefore, no probability can be assigned to an outcome. Leifer concludes:

" ... but I expect it can be made to work in other realist approaches to quantum theory, including those yet to be discovered."

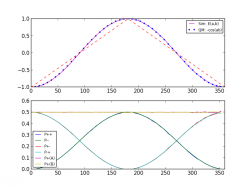
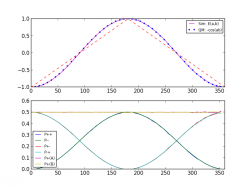
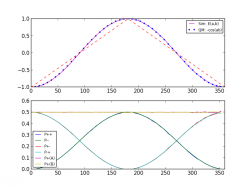
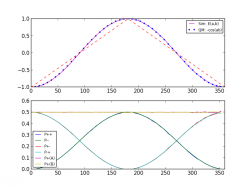
The only viable realist approach to quantum theory that I know of, that both forbids collapse of the wave function and fulfills the predictions of quantum measurement correlations demanded by standard quantum theory, is Joy Christian's measurement framework.

Very early on, I was concerned that Joy had sneaked Bayesian assumptions in by the back door -- which shows the extent to which even I have been indoctrinated -- in which case I would have dismissed the framework. It's clear now that no such probabilistic abracadabra infringes on the results.

The choice function of Bell-Aspect and CHSH type quantum experiments rests subjectively with the experimenter. The choice function in Joy's framework rests with random input to the continuous functions of nature independent of the experimenter. *That's* realism.

Tom

Joy, I get it. I truly do. Without deconstructing the opposing arguments, though, I don't see any way to let your new ideas shine through. One can eventually stop a fire after letting it burn itself out; however, we usually desire ways to rob it of fuel and oxygen, to save the precious assets in its path.

With one exception, I don't have an axe to grind with researchers who accept that reality is fundamentally probabilistic. They just haven't made the effort to learn otherwise.

Best,

Tom

The problem, really, is Bayes' Theorem.

It has no place as a guiding principle in the rationalist enterprise called science. It is just a tool for making inductively open judgements from phenomena, i.e., by Aristotelian method, rather than by the correspondence of logically closed judgments in scientific theory, to the natural world (Tarski, Popper).

To sacrifice truth to probability is not worthy of foundational science.

Let believers in Bell's theorem defend their choice with rational argument -- if they can find one.

Tom

Messed up that last link on the correspondence theory of truth.

Joy, this is just beautiful! My quick impression is that it may actually be experimentally replicable with electron input. It already reads like an experimental computation model.

Still nothing from the cone of silence where the critics are huddled?

(Great work by Michel Fodje!)

And I think that because the statistical function of Fodje's simulation verifies the topological structure of Roth's simulation, we can conclude, significantly:

The simulation of a continuous function is a continuous function.

Does it get more physically real and local than that?

I picked up a portion of a comment you made to a correspondent in sci.physics.foundations that I think is relevant:

"It is also important to realize that an orientation of a manifold (like that of a 3-sphere) is both a local and a global property at the same time. It is local in the sense that a basis is chosen at each point of the 3-sphere. But since this choice must be made consistently throughout the 3-sphere, its orientation is also a global (or a topological) property."

This local-global relation is an absolute breakthrough accomplishment, for future research directions -- including quantum computing without entanglement.

Yes, beautiful and impressive work for mathematicians. While not wanting to be a kill joy, it is only mathematicians who will insist that all lines and all planes, no matter their size contain the same number of points as my post on Nov. 5, 2013 @ 11:06 GMT refers.

Physicists, are now belatedly catching up with the idea that this is unlikely to be so. Einstein was already unto this long before as mentioned in a link. In a likely Quantum gravity theory there is likely to be a Planck limit at 10-35m. That being so, this line _____ and this _____________________ cannot contain the same number of points as Tom claims, since a Planck length cannot contain more than one point as it is indivisible.

I therefore stand to be corrected, that both Bell's theorem and its Disproof being founded on the assumption that there is no limit to distance at the Planck length will not be of much use to physicists standing at the gate between Quantum and Classical physics waiting and ready to "Tear down this wall"!

Akinbo

There is no wall, Akinbo.

"In a likely Quantum gravity theory there is likely to be a Planck limit at 10-35m."

What makes you think that?